Knot Again

In a previous post - Get Knotted - I worked out a design for a Celtic cross, used an iPad app to check that it worked and noticed that I could get the design to interlock by slightly overlapping the four units. Using the iPad saved me a lot of drawing, allowed me to mess around with the design, giving me the insight that I could overlap the units.

Int that what puters are for Awa Rich?

The next step was to figure out how to do this with traditional instruments. I'd worked out how to construct a unit in the 'Large Hadron Collider' schematic.

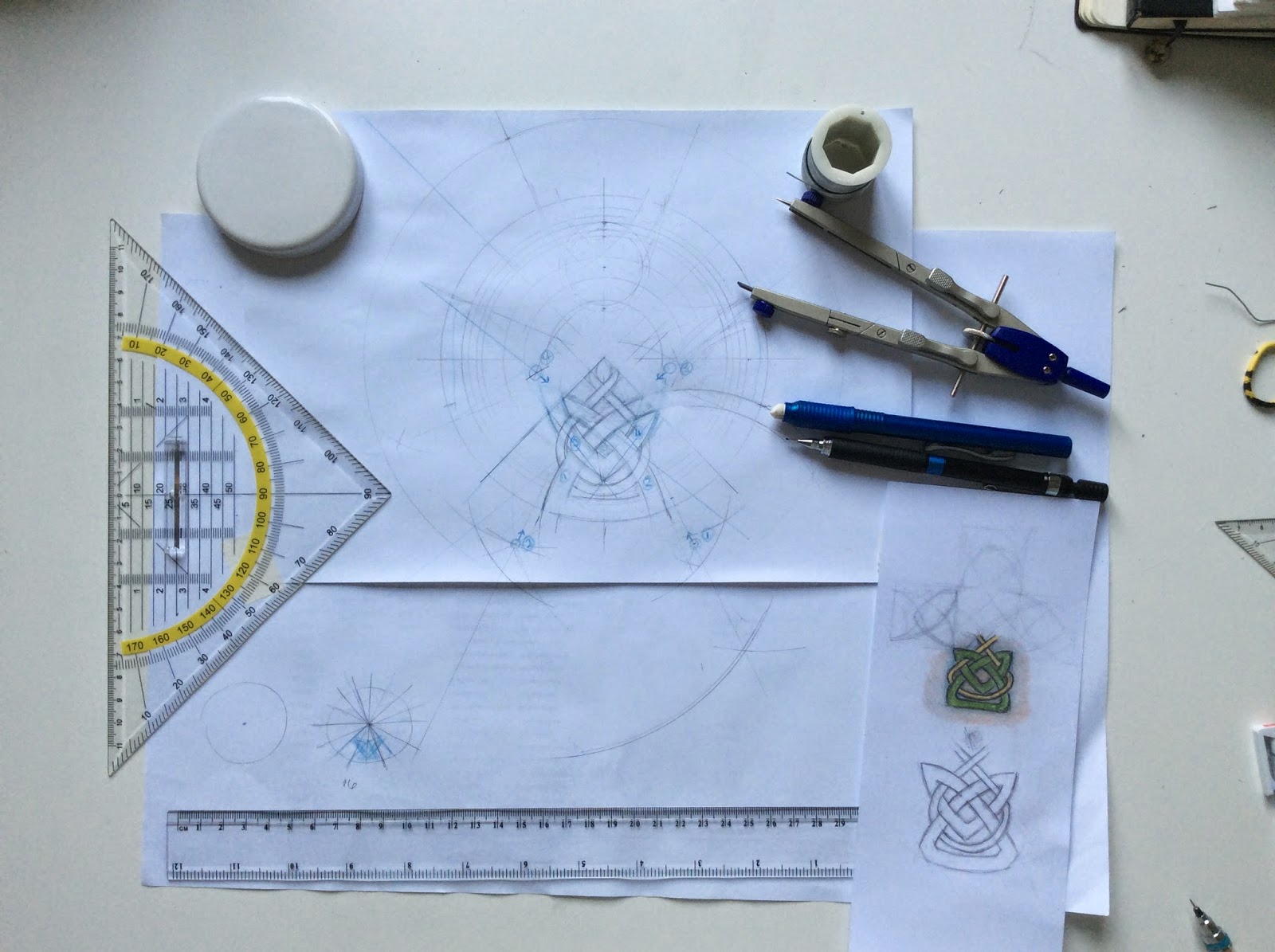The 'Large Hadron Collider' Schematic

Now all I had to do was repeat the process three more times. This was filling me with some trepidation due to the amount of chaos on the page caused by all the construction lines - which would need a lot of scrubbing out later.

I thought about cutting a cardboard template but that would take some doing and add another layer of inaccuracy. There had to be a way to simplify the construction using only the instruments you can see in the photo. Even so, I'm using technology that wasn't available to 9th century monks - nope, I'm not talking about the iPad - pencils, erasers and paper didn't become available until centuries later.

That's when the holes made by the compass point shone out like a little constellation - you can see I've circled them in the drawing so I could easily find them if I needed to redraw any lines. I already had my template, all I had to do was lay the drawing over a clean sheet of paper and prick through the holes to transfer them to the new sheet, then draw the arcs with the compasses. Rotate the template through 90 degrees and repeat. This proved to be a bit of a faff, it might have been easier to line up the dots if I'd used tracing paper.

It was rotating the template about the central hole that provided the next insight. I remembered technical drawing classes at school, how we were taught how to transfer measurements from one drawing to another using a pair of compasses. This method turned out to be a lot simpler and a clear pattern emerged.

It's amazing how something that seems horribly complicated at first, suddenly simplifies itself if you keep your eyes and brain open. Patterns reveal themselves like magic.

Sometimes you have to go the long way round to discover the shortcut Awa Rich!

Hmm, most sagely. I must write that one down.

Once the positions of the compass points were transferred using the compasses, it was a simple matter of drawing the arcs.

Another insight. I've been referring to each quarter of the drawing as a unit since the whole thing is the same pattern repeated four times rotated through 90 degrees. But notice that each arm of the cross is symmetrical about a line through the centre of the pattern. What I've been thinking of as a unit is actually two smaller units reflected about that line. The cake has 8 slices, not 4.

This reminded me of tedious, dry maths classes at school. Transformations - displacement, rotation, reflection and scaling - and here I was having fun with them.

Symmetry

This post is about a journey. Ideas, patterns, problems and insights that provided solutions - so no explanation of the step by step process is included. I'll do that another time.

Now all I have to do is colour it in.